高中数学-高考试题(全国统考 1983年复数的概念)

问答题（1983年全国统考）

当实数t取什么值时，复数z=+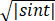i的辐角主值θ适合0≤θ≤π/4 ?

答案解析

因为复数z=+i的实部与虚部都是非负数，所以z的辐角主值θ一定适合0≤θ≤π/2.从而0≤θ≤π/4⇔0≤tanθ≤1.显然 r=|z|≠0.因为tanθ=sinθ/cosθ===,所以0≤tanθ≤1⇔0≤√(|t...

查看完整答案

讨论

高中数学

证明：对于任意实数t，复数z=+i的模r=|z|适合r≤.

计算行列式（要求结果最简）：

一个小组共有10名同学，其中4名是女同学，6名是男同学. 要从小组内选出3名代表，其中至少1名女同学，一共有多少种选法？

已知y=e-xsin2x，求微分dy.

在极坐标系内，方程ρ=5cosθ表示什么曲线？画出它的图形.

在同一平面直角坐标系内，分别画出两个方程y=-,x=-的图形，并写出它们交点的坐标.

设0.32,log20.3,20.3，这三个数之间的大小顺序是【】

设α=4π/3，则arccos⁡(cosα)的值是【】

三个数a,b,c不全为零的充要条件是【】

方程x2-y2=0表示的图形是【】

复数的概念

下面两个算式哪一个对？√(-4)∙√(-9)=2i∙3i=6i²=-6√(-4)∙√(-9)==√36=6

在复平面内，复数z对应的点的坐标是(-1,√3)，则z的共轭复数z ̅=【】

若z/(z-1)=i+1，则z=【】

在复平面内, 复数 z 对应的点的坐标是 (1, 2), 则 i · z =【】

已知 a ∈ R, 若 a − 1 + (a − 2)i (i 为虚数单位) 是实数, 则 a =【】

已知 i 是虚数单位, 则复数 z = (1 + i)(2 − i) 的实部是______.

已知复数z=+i，则arg 1/z是【】

求下列等式里x和y之值：(3xi+2x)+(yi-4)=(2y+5i)-(3+xi).

若对一切θ∈R，复数z=(a+cosθ)+(2a-sinθ)i的模不超过2，则实数a的取值范围为__________________.

设(1+2i)a+b=2i，其中a,b为实数，则【】

数系与复数

若ω为1之两立方虚根之一，试示=3

问ai=?(i=√(-1))

求1的三次根（实根和虚根），证：任一虚根的平方等于另一虚根，且((-1+i√3)/2)n+((-1-i√3)/2)n=-1，式中n为整数，唯不能为3的倍数.

(sinθ +icosθ)n = sinnθ +icosnθ.

已知a,b为正整数，a<b，且a,b互质.若关于x,y的不等式ax+by≤ab有且仅有2023组正整数解，则(a,b)=____________________(求出满足题意的所有可能数组).

设有理数r=p/q∈(0,1)，其中p,q为互素的正整数，且pq整除3600.这样的有理数r的个数为________.

给定正整数k(k≥2)与k个非零实数a1,a2,⋯,ak.证明：至多有有限个k元整数组(n1,n2,⋯,nk)，满足n1,n2,⋯,nk互不相同，且a1∙n1 !+a2∙n2 !+⋯+ak∙nk !=0.

设整数n≥4.证明：若n整除2n-2，则(2n-2)/n是合数.

Let m<n be positive integers. Start with n piles, each of m objects. Repeatedly carry out the following operation: choose two piles and remove n objects in total from the two piles. For which (m ,n) is it possible to empty all the piles?【译】设正整数m<n.起初一共有n 堆石子，每堆有 m块石子. 重复执行以下操作: 选择两堆石子，从这两堆中移除共n 块石子.问：对于怎样的 (m , n)，可以移除所有石子?

Consider an odd prime p and a positive integer N<50p. Let a1,a2,⋯,aN be a list of positive integers less than p such that any specific value occurs at most 51/100 N times and a1,a2,⋯,aN is not divisible by p. Prove that there exists a permutation b1,b2,⋯,bN of the a_i such that, for all k=1,2,⋯,N, the sum b1+b2+⋯+bk is not divisible by p.【译】已知奇素数p和正整数N<50p.设a1,a2,⋯,aN是一些小于p的正整数，同一数值至多出现51/100 N次，且a1+a2+⋯+aN不能被p整除.证明：存在a_i的一个排列：b1,b2,⋯,bN，使得对任意的k=1,2,⋯,N，都有b1+b2+⋯+bk不能被p整除.