△ABC 的内角 A, B, C 的对边分别为 a, b, c, 已知 cos2(π/2 + A) + cos A = 5/4.
(1) 求 A.
(2) b − c =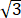 /3a, 证明: △ABC 是直角三角形.
/3a, 证明: △ABC 是直角三角形.
△ABC 的内角 A, B, C 的对边分别为 a, b, c, 已知 cos2(π/2 + A) + cos A = 5/4.
(1) 求 A.
(2) b − c =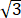 /3a, 证明: △ABC 是直角三角形.
/3a, 证明: △ABC 是直角三角形.
(1) 由已知得 sin2A + cos A = 5/4, 即 cos2A − cosA + 1/4 = 0. 所以 (cosA – 1/2)2 = 0 , cosA = 1/2.由于 0 < A < π, 故 A = π/3.(2) 由正弦定理及已知条件可得 sinB − sinC =/3si...
查看完整答案若 x, y 满足约束条件 , 则 z = x + 2y 的最大值是__________.
记 Sn 为等差数列 {an} 的前 n 项和, 若 a1 = −2, a2 + a6 = 2, 则 S10 = ______.
若 sinx = −2/3, 则 cos2x = _______.
设函数 f(x) = x3 − 1/x3 , 则 f(x)【 】
执行如图的程序框图, 若输入 k = 0, a = 0, 则输出的 k 为【 】
记 Sn 为等比数列 {an} 的前 n 项和. 若 a5 − a3 = 12, a6 − a4 = 24, 则 Sn/an=【 】
已知单位向量 a, b 的夹角为 60°, 则下列向量中, 与 b 垂直的是【 】
已知集合 A = {x| |x| < 3, x ∈ Z}, B = {x| |x| > 1, x ∈ Z}, 则 A ∩ B =【 】
于 A,B,C 三阵地测得敌机之仰角为 60°,45°,45°,今 B 地在 A 地正北 3000尺,C 地在 A 地之正西 4000 尺,求敌机之高,并讨论之.
堤上有塔高 50 尺,自堤下地面某点测得塔顶之仰角为 75°,塔底之仰角为 45°,求堤高.
△ABC 之底边 BC 的位置及长均为已知,自 B 至 AC 边之中线长亦为已知,求 A 点之轨迹.
已知在△ABC中,A+B=3C,2 sin(A-C)=sinB.(1)求sinA;(2)设AB=5,求AB边上的高.
已知在△ABC中,A+B=3C,2 sin(A-C)=sinB.(1)求sinA;(2)设AB=5,求AB边上的高.
已知a,b,c是△ABC中∠A,∠B,∠C的对边,S是△ABC的面积,若a=4,b=5,S=5,求c的长度.
记△ABC的内角A,B,C的对边分别为a,b,c,已知b2=ac,点D在边AC上,BDsin∠ABC=asinC.(1)证明:BD=b;(2)若AD=2DC,求cos∠ABC.
设二斜交轴 x 与y 交角为 θ,作一圆使通过 x 轴上之二定点 (a²,0),(b²,0)且与 y 轴相切,求此圆之方程式.
已知二圆C1:x²+y²-6x=0,C2:x²+y²-4=0,求通过C1,C2之两交点及另一点(2,-2)之圆的方程式.
求圆锥曲线 x² +y² = 49 及 x² +y² - 20y +90 =0之公切线的长.
一动圆与 (x - 2)² +y² =1及 Y 轴皆相切,求动圆圆心之轨迹方程.
若 kxy - 8x + 9y - 12 = 0 表示二条直线,求 k 值及此二直线所夹的角.
求自原点至圆x²+y²-14x+2y+25=0所作的二切线的交角.