已知在△ABC中,c=2bcosB,C=2π/3.
(1)求B的大小;
(2)在三个条件中选择一个作为已知,使△ABC存在且唯一确定,并求BC边上的中线长度.①c= b;②周长为4+2
b;②周长为4+2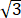 ;③面积为S△ABC=3
;③面积为S△ABC=3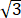 /4.
/4.
已知在△ABC中,c=2bcosB,C=2π/3.
(1)求B的大小;
(2)在三个条件中选择一个作为已知,使△ABC存在且唯一确定,并求BC边上的中线长度.①c= b;②周长为4+2
b;②周长为4+2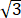 ;③面积为S△ABC=3
;③面积为S△ABC=3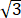 /4.
/4.
(1)由正弦定理b/sinB=c/sinC,得sinC=2sinBcosB=sin2B,故C=2B(舍)或C+2B=π.所以B=A=π/6.(2)由(1)知,c= b,故不能选①.选②,设BC=AC=2x,则AB=2 x,故周长为(4+2)x=4+2√3,解得x=1.从而BC=AC=2,AB=2,设BC的中点为D,则在△ABD中,由余弦定理,cosB=(AB2+BD2-AD2)/(2...
查看完整答案若P(cosθ,sinθ)与Q(cos(θ+π/6),sin(θ+π/6))关于 y轴对称,写出一个符合题意的θ值________.
已知a=(2,1),b=(2,-1),c=(0,1),则(a+b)·c=______;a·b=______.
已知抛物线C:y2=4x,焦点为F,点M在C上,且|FM|=6,则M的横坐标是______;作MN⊥x轴于N,则S△FMN=______.
数列{an}是递增的整数数列,且a1≥3,a1+a2+⋯+an=100,则n的最大值为【 】
已知圆C:x2+y2=4,直线L:y=kx+m,则当k的值发生变化时,直线被圆C所截的弦长的最小值为1,则m的取值为【 】
对24小时内降水在平地上的积水厚度(mm)进行如下定义:小雨:0~10中雨:10~25大雨:25~50暴雨:50~100小明用一个圆锥形容器接了24小时的雨水,则这一天的雨水属于哪个等级【 】
已知{an}和{bn}是两个等差数列,且ak/bk (1≤k≤5)是常值,若a1=288,a5=96,b1=192,则b3的值为【 】
某人在高处望见正东海面上一船首,其俯角为 30°,当船向正南行 a 里后,求得船首俯角为 15°,问此人之视点高出海面若干?
当△ABC 中A为钟角时,余弦定律为 a² =b² +c² +2bccosA.
设D为△ABC一边BC之中点,证AD²=1/4(2AB²+2AC²-BC²)
有等高的两竿,自其底连线上一点望之,较近之竿的仰角为 60°,若自该点向此线之垂直方向行 80 尺而测之,得二竿之仰角为 45°,30°,试求二竿之高及其间的距离.
试证三角形∠A之内角平分线之长为2bc∙cos(A/2)/(b+c).
堤上有塔高 50 尺,自堤下地面某点测得塔顶之仰角为 75°,塔底之仰角为 45°,求堤高.
设二斜交轴 x 与y 交角为 θ,作一圆使通过 x 轴上之二定点 (a²,0),(b²,0)且与 y 轴相切,求此圆之方程式.
若 kxy - 8x + 9y - 12 = 0 表示二条直线,求 k 值及此二直线所夹的角.
二直线x+y+4=0,x-y=0各与圆x²+y²-2x+4y-4=0相交,且所围成之二弓形面积相等,试证明之.
过一点 (2,1)的直线与直线 2x - 3y + 12 = 0 成45°角,求直线方程.
若三直线aix+biy+ci=0(i=1,2,3)相交于一点,则=0.试证之.
在定角 XOY 的二边上各取二点 P、Q,使 OP +OQ = a. 试求 PQ 的中点的轨迹.